Islamic Science and the Making of the European Renaissance

4. Islamic Astronomy Defines Itself: The Critical Innovations

Now that we have seen the kind of reactions the encounter with Greek science has produced in Islamic civilization, we can better appreciate the context for the astronomical developments that took place, as we continue to use astronomy as a template for the other disciplines that must have experienced similar transformations. In astronomy, the reactions expressed, at all levels, ranged from simple corrections of what was thought to be a mistake in the text, as was done by al-Ḥajjāj in the case of the Almagest, to correcting the basic parameters by fresh observations, as in the case of redetermining the better values of precession and the inclination of the ecliptic among others, to critiquing the methods of observation, as was done in the case of the fuṣūl method, and finally to casting doubt on the reliability of the very foundations of the Greek astronomical tradition itself when it seemed to violate the principles upon which it was based in the first place.

All these developments, when coupled with the very watchful eyes of the competing groups we spoke about earlier, from inside the profession as well as from outside, generated a skeptic attitude toward the incoming tradition. In itself this attitude emboldened astronomers to raise deeper and deeper questions as they continued to examine this Greek tradition in light of their own research. In this environment, it becomes easy to understand why good competent astronomers could not continue to practice astronomy by simply taking the Greek astronomical tradition at its face value. They had to compete by proving that they could achieve better results than those that were achieved by the Greeks and which were being continuously criticized at the time.

This did not mean that the Greek astronomical sources were yielding such dramatically erroneous mistakes that they could no longer be used to answer simple mundane questions like casting a horoscope or the like. But it did mean that the professional astronomer, from early Abbāsid times on, could no longer survive the competition if he limited himself to such simple questions in the first place. The serious astronomers had to answer more complex questions regarding the suitability of the proposed Ptolemaic astronomical configurations in accounting for the observations on the one hand, and in embodying the prevailing cosmological system of Aristotle on the other. For them, it was no longer sufficient to find the positions of the planets at any time for purposes of casting a horoscope or some such things, but they had to know how the planets moved, what caused their motion, why do they appear to go through all sorts of irregular behavior, and how does one account for that, all within the assumption of a universe made up of spheres all moving in place at uniform speeds as Aristotle had stipulated. At this degree of seriousness, Ptolemaic astronomy was found to be desperately wanting.

With the work of Muḥammad b. Mūsā b. Shākir, during the first half of the ninth century, regarding the properties and the admissibility of the existence of the ninth sphere, the stage was set for undertaking a total overhaul of the entire Greek astronomical edifice. When it was found later on, as we have already seen with the critiques of Ibn al-Haitham, that the physical foundations of the Ptolemaic configurations did not match the mathematical representations that were offered by Ptolemy, the motivation for the overall reform of that astronomy became a matter of necessity rather than choice. Only practicing astrologers could satisfy themselves, if they so pleased, with the use of the Ptolemaic Handy Tables, for example, to calculate the planetary positions that they needed for their horoscope casting. But those astrologers themselves fell under the censoring eye of the society at large, despite their ability to continue to function, and still try to make themselves useful to that society. Even then, they too had to require better and better astronomical tables (zījes) for their craft, as the old parameters of the Ptolemaic tables were continuously corrected as time went on. Socially though, no self-respecting astronomer would admittedly want to be cast in an astrologer's garb, if he could help it. This despite the fact that some of them did. While the best of them would want to associate themselves with the critical tradition that was beginning to pick up steam from the earliest decades of the ninth century. The latter had to cast a new name for the discipline they practiced (the discipline of 'ilm al-hay'a), because they did not wish to be associated with the lesser figure of the practicing astrologer.[235]

It was this environment that motivated the research of the new Islamic astronomy. Its main mission, as was enunciated later by Mu'ayyad al-Dīn al-'Urḍī (d. 1266) of Damascus,[236] one of the most distinguished astronomers of that tradition, was to create an astronomy that did not suffer from the cosmological shortcomings of Ptolemaic astronomy, that could account for the observations just as well as Ptolemaic astronomy could do if not better, and that did not limit itself to criticizing Ptolemy only, despite all the benefits that one derived from the detailed critique of Ptolemy's mistakes. This urgent need for a higher form of scientific astronomy was almost felt by all serious astronomers whose works we have come to know only recently, and who formed a continuous tradition inaugurated toward the beginnings of the ninth century and continued well into the sixteenth century as far as we can now tell. One astronomer after another would take very seriously Ibn al-Haitham's declaration which stated that there must be an astronomical theory, or in his language astronomical configuration (hay'a), that could account for the observations conducted in the real physical world without having to represent that world with a set of imaginary lines and circles as was done by Ptolemy.[237] One could hear them all repeat: If the world was real, made up of real spheres, as was argued by Aristotle, then let it be represented by mathematical models that did not contradict that physical reality.

On the more mundane level, when it was a matter of double-checking the observations that would account for the behavior of the real physical world, or that would help establish the very observations that were to be used as the building blocks of the theoretical representation, those had to be taken seriously as well. That is why one can document several attempts to double-check the values of the basic parameters, as we have already seen, or to initiate a whole discussion about the optimal methods of observation as we have also seen, or to initiate whole new fields of refining observational instruments or inventing whole new ones when there was a need for that. These activities continued to take place as the astronomical tradition continued to grow. The studies of Khujandī's surviving works on larger, and thus more precise, instruments, or those of 'Urḍī regarding the construction of the Marāgha observatory instruments, among others, speak exactly to such concepts.[238] But what was really wrong with Ptolemaic astronomy that generated all those discussions?

It is possible to say that Ptolemy saw the astronomical universe in four different ways: It was either a universe completely composed of Aristotelian spheres that could be described with the same language that was used in the Planetary Hypotheses, or a complementary world that was formed of those same spheres and represented by more precise predictive mathematical models as was done in the Almagest, or that it was a world that was already determined and its behavior tabulated as was done in the Handy Tables, or a world that was constantly at the mercy of the revolving celestial spheres that governed the world of change in the sublunar region in which we live, as was done in the Tetrabiblos.

For astronomers working in the Islamic civilization, the universe of the Handy Tables did not present much challenge, as it was a matter of fixing the mistakes of those Handy Tables by fresh observations whenever it was a matter of determining the positions of planets for any time and place. New parameters could do that, as was in fact done by generations of zīj writers who simply continued to update the Handy Tables. At times they added to them newer concepts that were not known in the Greek tradition that required tables of their own, such as tables for the visibility of the moon, or tables for prayer times, or qibla directions, etc., that were necessitated by the new religion of Islam and would not even occur to someone like Ptolemy. In such cases the newly established Islamic requirement of finding the best time and location for lunar visibility owed its inspiration to a religious practice rather than a scientific curiosity or astronomical need. And it is in such instances that religious thought would give rise to scientific thought and science could become a handmaiden of religion, as we shall see below.

The second Ptolemaic description of the world, that which was reflected in the Tetrabiblos, was quickly found to be way too general for use by the practicing astrologers. For although the Tetrabiblos gave a fairly sophisticated analysis of the manner in which the Aristotelian spheres and planets exerted their influence on the sublunar region, it did not offer detailed instructions on how to translate that theoretical analysis into practical horoscopes that could answer particular questions at specific times. For that reason more specific books had to be developed in order to make up for those shortcomings. Bīrūnī's book on the Elements of Astrology is a masterpiece in that regard,[240] as are the books of the various astrologers who attempted a more direct approach to the subject like the Introduction to Astrology of Abū Mā'shar.[241]

But the Ptolemaic books that caused the greatest amount of problems for the astronomers of the Islamic civilization were the Planetary Hypotheses and the Almagest. For although those two books were complementary to one another, yet they were mutually exclusive when it came to accounting for the Aristotelian cosmology in a more systematic fashion. From that perspective the Planetary Hypotheses spoke directly to a system of physical spheres, more or less in closer agreement with the Aristotelian spheres, while the Almagest spoke of circles representing spheres, and thus only implicitly acknowledged the Aristotelian spheres. Yet, both books spoke of physical impossibilities such as equants and the like. It was those impossibilities and absurdities that contradicted the Aristotelian cosmology that were found most objectionable.

It is not that Astronomers working in the Islamic civilization were enamored by Aristotelian cosmology and wanted to save it at any cost.[242] Rather it was that they saw in those two books clear indications of the Aristotelian assumptions about the composition of the universe and its constituent parts, and yet could not see the descriptive representations of that universe, as was done in the Almagest, really doing justice to the science of astronomy itself. When people read those two books, and they obviously read them together, as we have already mentioned at various occasions before, or when they read carefully the underlying assumptions as expressed in the Almagest too seriously, what they saw was a field that had accepted a set of Aristotelian cosmological premises, but went ahead and spoke about those premises in a language that contradicted their very essence. For instance, they saw Ptolemy speak about Aristotelian spheres as the constituent elements of the universe, and then turned around and represented those spheres with mathematical spheres whose properties would deprive them of their very sphericity. It was these kinds of fundamental contradictions that were thought of as detracting from the scientific basis of astronomy, and under no condition could serious astronomers accept those contradictions.

In what follows I only highlight the main features of these absurdities, and follow that with a description of innovative approaches that were taken by the astronomers of the Islamic civilization in order to emend, whenever possible, or create alternatives to the imported Ptolemaic astronomy.[243]

In the case of the sun, Ptolemy noted that if the observer were really located at the center of the Aristotelian universe, as the Aristotelian cosmology would require, then we would have routinely equal days the whole year round, we would have no seasons, and the sun would repeat its path around us day after day. But the observed reality was not like that. To account for that reality, Ptolemy first determined the basic parameters, like the length of a solar year, and then went ahead and proposed one of two solutions for the actual motion of the sun. He stipulated that the sun was either carried by an eccentric sphere, whose center did not coincide with the center of the Earth, as Aristotle would have wanted to insist, or that it was carried by another much smaller sphere — called epicycle — which itself was in turn carried by another sphere that was concentric with the Earth (figure 4.1).

In Book III of the Almagest, Ptolemy made sure first that both alternatives could still account for the observations well enough, and quickly resorted to the previous work of Apollonius (c. 200 B.C.) which in fact proved that both of these descriptions of motion could be represented by configurations that were mathematically equivalent in every respect. One did not have to chose, therefore, between them, if the purpose was only that of accounting for the observations.[244] In his own words "the mathematician's task and goal ought to be to show all the heavenly phenomena being reproduced by uniform circular motions,[245] and that the tabular form most appropriate and suited to this task is one which separates the individual uniform motions from the non- uniform [anomalistic] motion which [only] seems to take place, and is [in fact] due to the circular models; the apparent places of the bodies are then displayed by the combination of these two motions into one."[246]

Despite the fact that the actual motion of the sun could be represented in tabular form, both in terms of mean motion as well as in anomalisitic one, the problem still resided in the type of motions of the spheres that accounted for the observed motions. Obviously, Ptolemy's insistence on uniform motion here is undeniable. For he could not at the same time adhere to Aristotelian cosmology and yet allow any of the spheres to move at a varying speed as it pleased. And in case one forgets, in Book III, 3 of the Almagest, he made this uniform motion the general guiding principle of his astronomy in the following terms: "But first we must make the general point that the rearward displacements of the planets with respect to the heavens are, in every case, just like the motion of the universe in advance, by nature uniform and circular."[247]

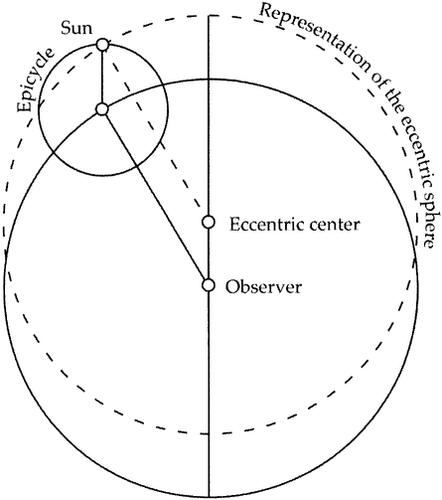
Figure 4.1

The equivalence of the eccentric and epicyclic models for the sun.

If Ptolemy was talking, as he seemed to be doing so clearly, about Aristotelian spheres that moved uniformly in place, then both alternatives that he proposed for the motion of the sun suffered from other Aristotelian considerations as we have seen before: First, the eccentric model of Ptolemy, would propose that there is a center of heaviness in the universe around which the most obvious luminary, the sun, would move, which was different from the Earth that was taken by Aristotle to be the center of heaviness par excellence. That is contrary to all the Aristotelian assumptions about the composition of the universe, and about the need to have an Earth at the center of the universe, not only as a center of heaviness to which all other elements would "naturally" gravitate or recede from, but as the fixed center of the sphere of the universe which is as essential to it as any fixed center is to a rotating mathematical sphere.

The second alternative, the epicyclic model, also assumed the existence of a sphere, out in the celestial realm, which had its own relatively fixed center of heaviness, different from that of the universe, and thus would make the element ether, of which all the celestial bodies are made, a complex element rather than the simple element Aristotle defined it to be.

These are the obvious contradictions that gave rise to the medieval discussions about eccentrics and epicycles, and about their undesirability in general, which we have already mentioned. And as we have already seen, they irked Ibn al-Shāṭir (d. 1375) of Damascus enough so that he would try to resolve them, as we shall repeat below. As for Ptolemy, and despite his insistence on the Aristotelian feature of uniform motion, he remained absolutely silent on these other Aristotelian considerations. In fact, he proceeded as if nothing was wrong and went ahead to assess the merits of each of the two models with respect to the criteria of simplicity. From that perspective he judged the eccentric model to be simpler on account of the fact that it involved one motion instead of two.[248] As far as he was concerned this instrumental reason was enough to allow him this temporary lapse of memory that there were other Aristotelian conditions to be met.

In fact, the situation became even worse as he proceeded. For although he could offer two options for the case of the solar motions, either an eccentric or an epicyclic one, when it came to the other planets he knew quite well that he would no longer have these options. He would have to use both eccentrics as well as epicycles in order to account for their more complex motions. Without any reference to Aristotelian cosmology, or any recollection that it was his guiding cosmology from the beginning, he went on to say: "... for bodies which exhibit a double anomaly both of the above hypotheses [meaning the eccentric and the epicyclic] may be combined, as we shall prove in our discussion of such bodies..."[249]

Although he had his own pedagogical reasons to do so, Ptolemy moved on to discuss the motion of the moon before discussing the motion of the other planets. It may be worth mentioning at this point that Ptolemy grouped the planets together in terms of the predictive mathematical model that he devised for their motions, of course with total disregard for Aristotelian cosmology as we just saw. As a result, he treated the motions of the sun, the moon, and mercury, separately and with separate models for each, and then grouped the other "upper" planets, Saturn, Jupiter, Mars, and Venus together and described their motion with one model. Furthermore, in the final arrangement of his presentation of the models, he also had to abandon the principle of simplicity and presented the models in the following order: sun, moon, upper planets, and mercury.

For the purpose of illustrating the underlying Aristotelian cosmological problems that these models entailed, I shall readopt the principle of simplicity and proceed to expose the problems with the model for the upper planets next, before I pass on to the models of the moon and mercury.

The motions of the upper planets Saturn, Jupiter, Mars, and Venus, described in Book IX of the Almagest and grouped together in IX, 6, can be briefly summarized in the following manner (figure 4.2): Each of the upper planets was supposed to be carried by an epicycle, attached to it in the same fashion a crown is attached to and carried by the ring, to use medieval descriptions.

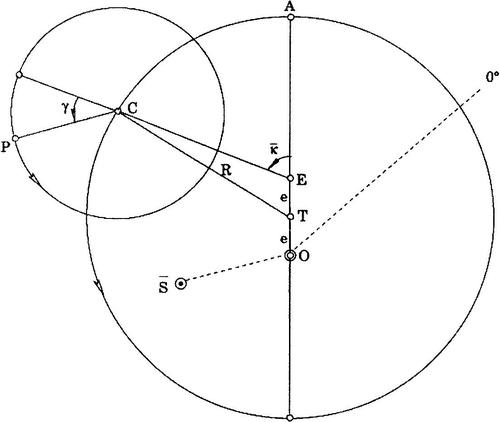
Figure 4.2

Ptolemy's model for the upper planets. The observer is at point O. The planet P is carried on an epicycle with center C, which is in turn carried by the deferent with center T. Note that the deferent rotates uniformly around the equant E and not around its own center T.

The epicycle was itself carried within the shell of and by an eccentric sphere called the deferent, here represented by a simple circle with center T. Each of those spheres moved uniformly in order to account for the anomalistic and mean motions respectively. But in order to account for the observations properly Ptolemy had to assume that the carrying deferent sphere did not move uniformly around its center T, nor around the Earth which was at the center O of the universe still, but around another point E, called later the equant point, or the center of the equalizer of motion when described by Ptolemy as a sphere. Without any proof of any sort, Ptolemy went on to stipulate that the equant point was located away from the deferent's center, by the same distance as the center of the deferent itself was removed from the center of the universe, and on the opposite side. That is the eccentricity OT was equal to TE.[250] With this arrangement, the deferent's uniform motion around its equant accounted for the mean motion of the planet, and the epicycle's motion around its own center accounted for the anomalistic motion, and thus the phenomena were sufficiently saved.

But from the Aristotelian perspective, this new predictive model for the positions of the planets did not only violate the Aristotelian presuppositions once with the adoption of the eccentric sphere, but twice with the adoption of the epicyclic one as well. It even went beyond that to violate, in a very serious fashion, the very mathematical property of a sphere. With Ptolemy's new assumption that there could be a physical sphere that could move uniformly, in place, around an axis that did not pass through its center, it became very clear that the same assumption would require us to abandon completely the very concept of the mathematical sphere and its defining properties. The only axis around which a physical sphere could move uniformly in place was the one that had to pass through the fixed center of the sphere; otherwise it could not stay in place.

Even if Ptolemy could have satisfied the Aristotelian conditions by avoiding the eccentric sphere and accounted for it by another epicycle, as he did with the case of the sun, and even if he had allowed himself the license to use epicycles, arguably against the Aristotelian conception of the simplicity of the element ether, as was done later by Ibn al-Shāṭir of Damascus (1375), for example, still his requirement that any physical sphere could move uniformly around an axis that did not pass through its center would make the existence of such a sphere physically impossible. And as was clearly stated by Ibn al-Haitham later on, and already quoted before, we do not live in an imaginary world where such spheres may only exist in the mind, but in a very real one whose motions had to be accounted for.

On the positive side, Ptolemy's configuration, physically absurd as it was, could still account rather well for the longitudinal motions of the planets. It could explain the daily progression of the planets from west to east, i.e. contrary to the direction of the primary daily motion of the heavens, and in the direction of the ascending order of the zodiacal signs Aries, Taurus, Gemini, etc., as a result of the planet's own mean motion. The planet's particular motion, said anomalistic motion, took place uniformly on its own epicycle, and could account for the forward and backward motions of the planet as well as account for the stations in between. These positive conditions, when coupled with the ability of the model to predict the positions of the planets rather accurately, for its time, could satisfy the astrological predictions and the like.

On the negative side, the sheer absurdity of the equant concept, in the realm of physical spheres, turned this model into a point of contention to be taken up by every serious astronomer up to and including Copernicus.[251]

In the case of the moon, Ptolemy's model became more complicated, and even more absurd than the two previously discussed models of the sun and the planets. In order to account for the observable motion of the moon, with its variations in the position of eclipses, the apparent motion of the moon on its epicycle without undergoing retrograde motion, and the variation in the size of the epicycle as it appears to the observer on Earth, he could not possibly account for all those variables with a relatively simple model as that of the sun or the planets. Instead he introduced the "sphere of the nodes" (figure 4.3) as an engulfing sphere for the moon and made it concentric with the Earth. He made this sphere responsible for the motion of another sphere inside it that he called the deferent, which was in turn eccentric with respect to the Earth. He made the sphere of the nodes move from east to west, while the engulfed deferent in the opposite direction. The moon was finally moved directly by an epicycle which was carried within the shell of the deferent but which moved in the direction opposite to that of the deferent.

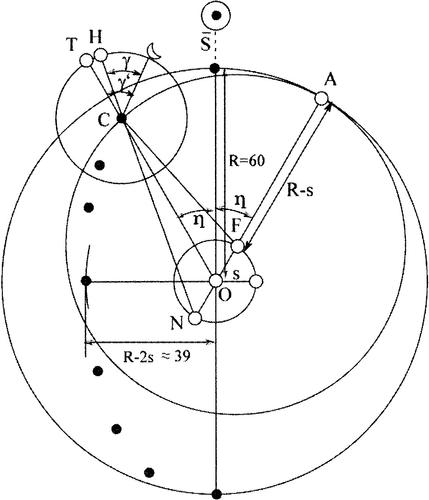
Figure 4.3

Ptolemy's model for the moon where the engulfing sphere that moves the nodes as well as everything contained in it is centered on the Earth at O. The deferent sphere, with center F, is moved by the engulfing sphere from east to west so that its apogee moves to point A. The deferent that carries the epicycle within its shell, here represented by the circle of the deferent itself, moves in the opposite direction to bring the center of the epicycle to point C. Note that the uniform motion of the deferent is measured around the center of the universe O, which means that it does not move uniformly around its own center F, thus producing an equant-like concept of its own. Now the epicycle moves by its own anomalistic motion in the same direction as the engulfing sphere. But its anomalistic motion is measured from the extension of the line that connects the center of the epicycle C to the ever-moving prosneusis point N. Furthermore, note that the distance of the epicyclic center from the Earth, when the epicycle is 90° away from the mean sun is almost half the distance it would have when the epicyclic center is in conjunction or opposition with the sun. That means that a quarter moon should look almost twice as big as a full moon, which is obviously untrue.

But in order to create a variation in the size of the epicycle, especially when the moon was away from the mean sun by 90°, Ptolemy made the deferent move uniformly around the center of the Earth rather than around its own center, thus creating again an equant problem similar to that encountered with the upper planets. Furthermore, and in order to account for the second anomaly of the moon, he stipulated that the moon's own motion on its epicycle should be measured from a line that started at a point, N in the diagram, which was located diametrically opposite from the deferent center, F in the diagram, with respect to the Earth, and at the same distance from the Earth as the deferent center, but in the opposite direction. The point was called the prosneusis point (nuqṭat al-muḥādhāt) and the line joining it to the center of the epicycle, when extended to the circumference of the epicycle, constituted the starting point for the true anomaly of the moon. One should note here that this prosneusis point N was itself in constant motion as it was always defined by its diametrically opposite location from the moving center of the deferent F. That is, it was simply symmetrical to the deferent center with respect to the center of the universe. Thus the line that joined the prosneusis point to the center of the epicycle oscillated back and forth from the line that joined the center of the epicycle to the fixed center of the universe and never completed a full revolution. This oscillating motion was found objectionable as well, since there should not be any non-circular motions in the heavens according to Aristotle, and thus all revolutions should be completed.

For very instrumental reasons, this crank-like model, however, accommodated the observations rather well, as far as the longitude of the moon was concerned. But it failed miserably when it came to the apparent size of the moon. If taken seriously, and with its crank-like operation, the model required the moon to be pulled very close to the Earth when it was at 90° away from the mean sun. Accordingly, the moon's distance from the Earth at that point would become almost half the distance it had when it was at full moon or in conjunction with the sun. This would mean that for an observer, situated on the Earth, when the moon was quarter moon, it would then have to appear twice as big as when it was a full moon. This predictive aspect of the model was obviously untrue, and was fittingly described later on by Ibn al-Shāṭir as untenable since the moon was never seen as such (lam yura kadhālika).

Furthermore, since the position of the prosneusis point itself was determined by the position of the symmetrically opposite position of the deferent center on the other side from the Earth, and since that center was itself moved around the Earth by the engulfing sphere of the nodes, that meant that both the deferent center as well as the prosneusis point, that depended on it, were in constant motion. This also meant that the line that joined the prosneusis point to the center of the epicycle's center was no longer fixed as well as we just said, and thus in 'Urḍī's terms, was not fit to be considered the beginning line for the measurement of the anomalistic motion, since it would not constitute a fixed starting point.

With a deferent that moved around its own center, but measured its uniform motion around another center, now the center of the universe, thus repeating the same equant problem, and with the introduction of the moving prosneusis point that introduced an oscillating line that never completed a full revolution, and with the huge increase in the apparent size of the moon at quadrature, all implied by Ptolemy's model, one can see why this model attracted a large critical literature within Islamic civilization. Its own problem was often referred to as the prosneusis problem, in analogy to the equant problem that was used to describe the difficulties with the model for the upper planets. Several astronomers working in Islamic civilization tried to rectify the situation by creating their own models, some of which were more successful than others. In that tradition, Ibn al-Shāṭir's lunar model was by far the best, not only because it did away with the equant construction when it made all spheres move uniformly in place around axis that passed through their own centers, and reduced the variation in the apparent size of the moon, while keeping the increase of the size of the epicycle, but because it also turned out to be identical to the same model which was proposed by Copernicus (d. 1543) himself about 200 years later.[252]

In his usual fashion, Ptolemy said nothing about the difficulties of his model, and did not even draw attention to the fact that his model directly contradicted the real apparent size ever so blatantly. But things were moving from worse to worst, as the next model of Mercury and the models for the latitudinal motions of the planets were worst still.

Because of the high speed of this planet, and because of its proximity to the sun, and thus the difficulty in observing it in a reliable fashion, Ptolemy's model for the motions of this planet reflected the faulty conditions of the observations.[253] As in the case of the moon, where Ptolemy's crank-like model predicted that the moon would come closest to the Earth twice during its monthly revolution (when the moon reached 90° or 270° from the mean sun, or when the moon was at the first or third quarter of its revolution), so was the case with Mercury, which was supposed to come closest to the Earth at two points during its revolution: when Mercury was 120° away from the apogee, on either side of the apsidal line. This meant that Ptolemy's model for Mercury would mimic some of the features of the lunar model.

In Almagest IX, Ptolemy proposed a model for the planet Mercury that had an engulfing eccentric sphere called the director (centered at B in figure 4.4), which in turn carried another eccentric sphere called the deferent (here centered at G). Needless to say, both eccentrics were in direct violation of the Aristotelian presuppositions. The director moved around its own center, in the direction opposite to the succession of the signs, i.e. from east to west, and carried with it the deferent in the same direction. The deferent, however, moved in place, inside the director, by its own motion but in the opposite direction, thus producing a crank-like mechanism that was similar to that which was employed in the lunar model. And like the lunar deferent, this one too did not move uniformly around its own center G, but around a center E, also called the equant as in the model of the upper planets, but placed, again without any proof, half way between the center of the Earth and the center of the director, instead of being on the far side as was the case with the upper planets. The epicycle, which carried the planet Mercury with its own anomalistic motion, moved in the same direction as that of the deferent, and was itself carried by the motion of the deferent in the direction of the succession of the signs.

Thus, in addition to two eccentrics (which one may have thought that Ptolemy could explain away in the same way he used the Apollonius theorem to explain the solar eccentric away) and one epicycle (unavoidable on account of the second anomaly), there was the same additional absurdity which had appeared twice before: the absurdity of having a sphere move uniformly, in place, around an axis that did not pass through its center. And as in the case of the model for the upper planets, there was the additional unproved statement of Ptolemy that the equant laid half way between the center of the world and the center of the director. One can see why such accumulated technical considerations would make Ptolemaic astronomy subject to the kind of severe criticism that was leveled against it once it came into Islamic civilization.

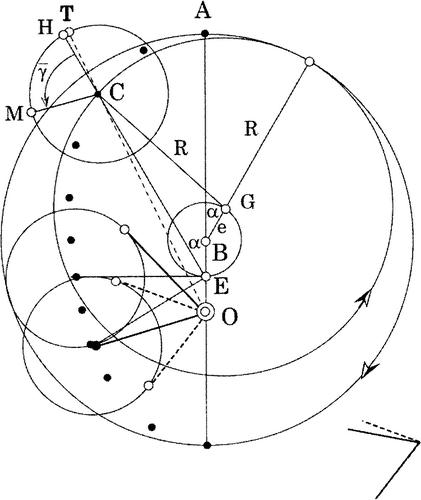
Figure 4.4

Ptolemy's model for Mercury. The observer is at point O, the center of the universe. The planet M is carried by the epicycle with center C, which is itself moved by the deferent with center G. The deferent, which is also moved by an engulfing sphere called the director and whose center is B, moves in the same direction as the epicycle, but measures its equal motion around the equant E rather than its own center G. The equant E is halfway between the center of the universe O and the center of the director B. For the observer at point O, Mercury's epicycle will appear at its largest when it is closest to Earth, at ±120° away from the apogee A, and not at quadrature, when it is only 90° away from the same apogee, as was thought by Copernicus. The two elongations are represented here by angles drawn with dotted and continuous lines.

To make matters worse, the Ptolemaic models for the latitudinal motion of the planets further introduced some absurdities of their own. In this instance, and for purposes of computing the latitudinal component of the planetary positions, Ptolemy made a distinction between two groups of planets: He grouped Saturn, Jupiter, and Mars in one group and described their latitudinal motion with one model, and grouped Venus and Mercury in another group that was the subject of a different, and quite offending, model. It should be stressed at this point, that in terms of longitudinal motions the models described by Ptolemy still yielded quite reasonable predictive results despite their physical absurdities. Those results were at least convincing enough to allow Ptolemy to make his pragmatic claim that he must have been following some correct conjecturing in configuring them out although he did not know rigorously enough how they worked.

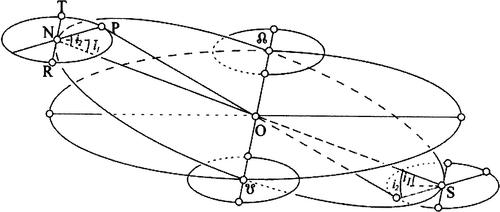
Figure 4.5

Ptolemy's model for the latitude of the upper planets. The observer is at point O, and the inclined deferent plane has a fixed inclination. The epicycle however had its own deviation from the deferent plane, whose value depends on the position of the epicycle along the deferent.

For the upper planets (figure 4.5), Ptolemy proposed a model that included an observer at the center of the world O, that is the center of the ecliptic. He then proposed an inclined, eccentric deferent plane that intersected the plane of the ecliptic at a fixed angle. The line of the intersection between the two planes passed through the position of the observer and marked the two nodes. The epicycle that was carried by the inclined plane had its own deviation from that plane as well, but this deviation varied depending on the longitudinal position of the epicycle. At the northernmost end of the deferent the epicycle would have its maximum deviation, but as soon as the epicycle reached the position of the nodes it would lie flat in the plane of the ecliptic. At the southern end of the deferent it will have the same phenomenon of maximum deviation, but in the opposite direction. And although both deviations had the same value, the southern one simply looked bigger since it was closer to the observer.

In effect, then, the plane of the epicycle seemed to perform a seesawing motion of its own, as the position of the epicycle changed by the motion of the deferent. Since this kind of motion never completed a full circle, it was obviously deemed to be in the same category as the oscillating prosneusis of the moon. And thus it was as far as it could be from the uniform circular motion, which Aristotle would have required, since only full circular motions were the natural motions of the simple element ether of which all the celestial bodies were composed.

No word from Ptolemy in this regard. And although he had claimed that he was adhering to the Aristotelian cosmology, he still behaved in the same fashion as he did before when such violations were committed. That is, he still made no effort to explain them away as one would have expected. Instead, he invited the reader to imagine that the tips of the epicyclic diameters could be attached to pairs of "small circles." Those circles could be placed perpendicular to the plane of the deferent. And as the tips of the diameters moved along those "small circles" the resulting oscillating motions would produce the seesawing effects that were needed. He then had a problem synchronizing those motions of the tips of the diameters along their "small circles" and the motions of the epicycles themselves along their deferents, since the deferents themselves were eccentric, as we have already seen. To solve the problem, he resorted once more to the assumption that the diameter tips too had their own form of equants just like those of the other larger models of the planets, since they did not seem to be partaking of a uniform circular motion around their own centers.

Now, even if one could accept the motion of the diameter tips for the purposes of producing the seesawing effect, which was in turn "justifiably" required by the observations, one could easily see that any such "unnatural" seesawing would also create a wobbling effect that would interfere with the longitudinal component for which much pain was suffered when it was computed in the first place.

It was this specific feature of the latitudinal motion in the Ptolemaic model that led the thirteenth-century astronomer Naṣīr al-Dīn al-Ṭūsī (d. 1274) to proclaim, in his Taḥrīr al-majisṭī in 1247, that Ptolemy's speech was indeed intolerable, or, in his own polite words, beyond what was permitted in the craft (khārij 'an al-ṣinā'a).[254]

The latitudinal motion of the second group, the lower planets Mercury and Venus, did not fare any better in this regard. For them the inclination of the carrying plane itself varied as the epicycle moved along its circumference. One should always remember that those planes were supposed to be the equators of physical spheres, the only bodies capable of generating such motions in an Aristotelian universe. In the case of Venus, when the epicycle was at the northernmost end of the inclined plane that plane itself tilted northwards and the epicycle on its northern edge tilted away from the carrying plane along the eastern edge. But as the epicycle moved to the nodes one of its diameters coincided with the ecliptic, while the other still tilted along the eastern edge. When the epicycle reached the southernmost end, the whole inclined plane tilted in the opposite direction so that its southern end now pointed to the north, and the epicycle still inclined away from it along the eastern edge again. In effect then both the inclined plane as well as the plane of the epicycle itself would undergo the same kind of seesawing motion that was noticed in the model of the upper planets. And here again, the only solution Ptolemy had to offer was to propose the same kind of "small circles" to be attached to the seesawing diameters so that they could be forced to perform the latitude motion. And here again that arrangement would still force the whole plane to wobble and destroy the longitudinal component as before. We just saw what Ṭūsī would say of such an arrangement.

In sum, Ptolemy's models for the movements of the moon and the five planets introduced notions that were not only in violation of the Aristotelian presuppositions, but as we have seen, with the case of the motions in latitude of the planets, included arrangements that also destroyed the longitudinal components that worked rather well on their own. It looked like Ptolemy could not compute any component of the motion without destroying the other. In total exasperation, he ended up confessing that only gods were capable of such perfection, not the mere humans.[255] With this realization, the whole Ptolemaic configuration seems to fall apart, despite the fact that, on the computational level, it seems to have been able to predict the positions of the planets, and can still do, with a rather remarkable accuracy.

The reforms of this astronomy that were to take place in Islamic civilization after the thirteenth century went to great pain to retain that predictive value of Ptolemaic astronomy. But they definitely aimed to reform the conceptual arrangements of the spheres that were supposed to carry out these various motions. Toward the end of the twelfth century, when Averroes had to give his assessment of the Ptolemaic astronomy, an astronomy that was still the norm in his time, he had the following to say: "The science of astronomy of our time contains nothing existent (lays minh^u shay'^un maujūd), rather the astronomy of our time conforms only to computation, and not to existence (lā li-l-wujūd)."[256]

We have already seen several levels of responses to what was perceived as factual mistakes in the Ptolemaic tradition. Whether it was in simple mistakes in texts, or basic parameters, or even methods of observations, those were attended to and began to be fixed as early as the ninth century. New genres of writings addressing specifically the totality of those Ptolemaic problems, called shukūk, istidrāk, and the like were developed and sophisticated with time, so much so that they became subjects of discussion on their own by people who were not even astronomers by profession.

Serious attention to the philosophical and physical underpinnings of the Ptolemaic edifice, otherwise signaled as model building, and serious attempts to replace the inadequate Ptolemaic models did not begin until the eleventh century. But once it began, almost every serious astronomer felt that he had to take part in the enterprise. In the sequel I will only signal those who made fundamental shifts in the way astronomy was practiced to the neglect of others who kept the discipline alive by supplying the commentaries and the individual modifications that they saw the major shifts required, or simply made use of those shifts to overhaul the then current astronomy in order to incorporate those changes.[257] For example, when the trigonometric functions were introduced into the Islamic scientific tradition, and were perfected after being originally derived from the few functions already known in India, the tendency was to use those functions in any theoretical discussion of astronomy instead of the chord functions that were used in the Almagest and its translations.

It is these kinds of shifts that produced the astronomy that could then be called Arabic/Islamic astronomy, and whose example we hope the other disciplines had followed. In what follows, however, I will pay a special attention to the most subtle shifts that played, in my judgment, a catalytic role in producing other astronomical innovations, and became part of the universal legacy of astronomy. As was already said, I will neglect those who periodically took in those conceptual shifts and integrated them in their works without producing any shifts of their own.

The focus will then be on those astronomers who felt that they needed to invent new concepts, or more concretely new mathematical theorems, in order to solve the problems of Ptolemaic astronomy, and not that much on those who followed them by incorporating the latest theorems to build upon them in order to create new planetary models of their own. Of those who introduced such new theorems, the names of Mu'ayyad al-Dīn al-'Urḍī (d. 1266) and Naṣīr al-Dīn al-Ṭūsī (d. 1274) stand out on account of the fact that each of them supplied his own mathematical theorem while undertaking to overhaul the fundamental features of Ptolemaic astronomy.

'Urḍī thought that Ptolemy's models for the sun were adequate enough, and that Ptolemy's choice of the simple eccentric model was innocuous enough, that it did not deserve any special transformation. Neither 'Urḍī nor Ptolemy ventured to state explicitly what he really thought of the epicyclic model, posited by Ptolemy at least as an alternative to the "offensive" eccentric model. One suspects that the ubiquitous use of epicycles in all other planetary models, and the impossibility of their replacement, may have made their use a necessity that could not be avoided. But no one was willing to defend the use of those epicycles explicitly. Their final theoretical solution would not come until a century later with the works of Ibn al-Shāṭir (d. 1375) as we shall soon see.

As for the motions of the moon and Mercury, and the notorious equants in both models, in addition to the prosneusis point in the case of the moon, 'Urḍī felt that he could not let things be. Instead he decided to take advantage of the similarities between the two models, and tried to reconfigure them by adopting three new steps. First he decided to shift the directions of the motions of the various spheres. Then he adjusted the magnitudes of those motions. And finally he tried, in a global way, to avoid the Ptolemaic handicaps that plagued both models by making all spheres move uniformly on axis that passed through their centers. At this point he still restricted himself to the mathematics that was available to Ptolemy from Euclid's Elements, for example, without having to offer any new mathematical propositions of his own. There were times though when he would venture to say that he faulted Ptolemy for his inability to theorize (ḥads) properly, but would still express his full admiration for Ptolemy's observational and mathematical control of the data. Such shifts in theorizing, as long as they did not involve the introduction of new material like the trigonometric functions, were quietly introduced nevertheless without much fuss.

But when it came to the model of the upper planets, 'Urḍī felt that the Ptolemaic model was no longer redeemable, and thus had to be reconfigured in a fundamental way. It was there that he proposed to introduce what has now become known in the literature as 'Urḍī's Lemma in order to resolve the very thorny issue of the equant problem. At this point, 'Urḍī's concern was no longer focused on the cosmological choices of eccentrics versus epicyclic models, but was focused on the more fundamental equant stipulation which forced the very sphere that was supposed to carry out the motion of the epicycle to loose its sphericity. This physical impossibility could not be tolerated, and still pretend to carry out astronomical theorizing, as these astronomers saw their functions to be. Instead, 'Urḍī approached the problem of the model of the upper planets with the mathematically rigorous manner it deserved.

After demonstrating the physical failings of Ptolemy's model, he went on a tangent and said that in order to theorize better about the motions of those planets, he needed to introduce a new theorem, the statement of which could be rephrased thus: Given any two equal lines that form equal angles with a base line, either internally or externally, the line joining the extremities of those two lines would be parallel to the base line.[258]

Taken on its own, 'Urḍī's Lemma looked like a generalization of Apollonius's theorem, in that the equal angles needed for the proof of the parallelism of the end line with the base line are no longer restricted to the exterior angles used in the construction of the epicyclic model. Instead 'Urḍī could show that the internal equal angles would produce the same effect of parallelism and thus could be used to rectify the instance of the equant without losing its observational value that had forced Ptolemy to adopt it in the first place.

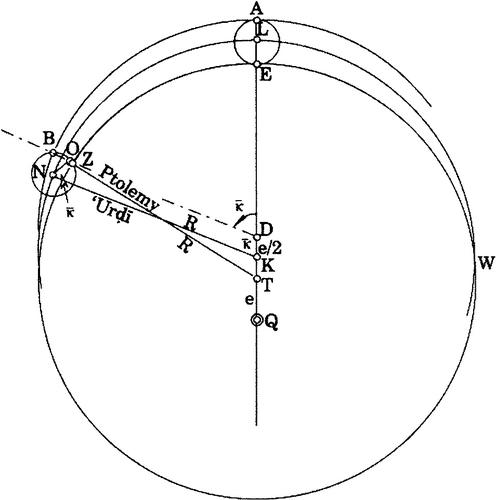
Figure 4.6

'Urḍī's model for the upper planets. By defining a new deferent with a center at K, halfway between the center of the Ptolemaic deferent T and the equant D, 'Urḍī allowed that deferent to carry a small epicycle whose radius was equal to TK = KD. He made the small epicycle move at the same speed as the new deferent, and in the same direction. By applying his own lemma, 'Urḍī could demonstrate that line ZD, which joined the tip of the radius of the small epicycle to the equant, would always be parallel to line KN, which joined the center of the new deferent to the center of the small epicycle. He could also show that point Z, the tip of the radius of the small epicycle, came so close to the point O, which was the center of the Ptolemaic epicycle, that the two points could not be distinguished. Then it was easy to see that the uniform motion of O that Ptolemy thought took place around point D was indeed a uniform motion around point N which in turn moved uniformly around K, thus making Z appear to be moving uniformly around D and satisfying the Ptolemaic observations.

Instead of assuming (figure 4.6) that the epicycle is carried by a deferent that moved uniformly around an axis that did not pass through its center, as was done by Ptolemy, 'Urḍī shifted the center of his new deferent to a point K, which was located halfway between the center of the old Ptolemaic deferent T and the equant point D. He then allowed this new deferent to carry a small epicycle whose radius was equal to half the Ptolemaic eccentricity, or equal to the same magnitude by which the center of the deferent was shifted in the first place. The small epicycle moved at the same speed as the old Ptolemaic deferent, and in the same direction, and in turn carried the Ptolemaic epicycle. The combination of the equal motions allowed the lines joining the extremities of the small epicycle's radius to points K and D respectively to be always parallel. This made the center of the Ptolemaic epicycle, now carried at the extremity of 'Urḍī's small epicycle, look like it was moving uniformly around the Ptolemaic equant. In fact it moved around the center of its own small epicycle, and the center of that epicycle, in turn, moved around the center of the new deferent. With all spheres now moving uniformly, in place, around axis that passed through their centers, 'Urḍī managed to avoid the absurdity of the Ptolemaic equant altogether, and, at the same time, still retain its observational value, as was required by the Ptolemaic observations.

'Urḍī's Lemma, introduced through the mechanism of the small circle in the model for the upper planets, proved to be a very useful tool for other astronomers and at other occasions as well. A whole host of astronomers ended up using it in order to construct their own alternative models to those of Ptolemy. Astronomers such as Quṭb al-Dīn al-Shīrāzī (d. 1311) used it in his lunar model. While Ibn al-Shāṭir of Damascus (d. 1375) ended up using it in more than one of his own models. 'Alā al-Dīn al-Qushjī (d. 1474) used it in his model for the planet Mercury, and Shams al-Dīn al-Khafrī (d. 1550) made a double use of it in his own model for the upper planets. Finally, Copernicus (d. 1543) used it for the same model of the upper planets. As it turned out, this mathematical tool became very fecund in the construction of all sorts of responses to Greek astronomy.

From that perspective, this relatively simple lemma proved to be an epoch maker, just like the Ṭūsī Couple, which will be mentioned later. And like the Ṭūsī Couple too, once it was discovered, it allowed several generations of astronomers to think differently about Ptolemaic astronomy, and about the possibilities with which this astronomy could be reformed.

As far as 'Urḍī was concerned, it turned out that with one new theorem, and with small adjustments to the directions and magnitudes of motions, he could reconfigure the whole body of Ptolemaic astronomy, and still produce his own configuration that was free of the absurdities of the equant and the like. In that regard he ended up playing a pivotal role in the development of Arabic astronomy, a role comparable only to that of Naṣīr al-Dīn al-Ṭūsī (d. 1274) as we shall soon see. His work on the planetary latitudes, however, like the work of all the other medieval astronomers, up to and including Copernicus, it could not resolve the major issues with Ptolemaic astronomy as elegantly as it could solve the longitudinal component.

'Urḍī's former boss at the Marāgha observatory came to his own solution of the equant problem in a slightly different fashion. To him, the problem was not fundamentally a problem of an epicycle that moved uniformly around an equant point, thus creating the physical absurdity, but was more a problem of a uniform motion that was observed from varying distances thus appearing to be non-uniform. One way of thinking about it was to allow the center of the epicycle in the model for the upper planets to move uniformly while at the same time still allow it to draw close to the point of Ptolemy's equant when close to the apogee, and move away while at perigee. This motion would in effect duplicate the phenomenon that Ptolemy said was exhibited by the observations. Therefore one could solve the problem if he/she could devise a way in which a body moving in uniform circular motion could still be allowed to come close to a specific point and draw away from it while at the same time retain the uniform circular motion undisturbed. The net effect would be that the body would be perceived to move at varying speed in an oscillating motion with respect to that point when, in fact, it would in itself continue to move in uniform circular motion. The problem was to achieve an oscillating motion in the realm of spheres that were all supposed to move uniformly around their own centers.

The idea of an oscillating motion resulting from circular motion seems to have occurred to Ṭūsī when he was tackling the problem of the Ptolemaic latitude theory. This was apparently the same circumstance under which Copernicus reached the same connection between the two phenomena.[259] Later on in the Commentariolus, and while describing the motions of the planet Mercury, Copernicus goes further by clearly making reference to the second connection between the motions of Mercury and the motions in latitude. At that point he does in fact describe the same Ṭūsī Couple that he used for his own Mercury Model as being related to the motions that he had already described in the latitude theory.[260] This very connection between the genesis of the Ṭūsī Couple and the motion in the latitude theory first came about when Ṭūsī had already noted, some three centuries before Copernicus, in his Taḥrīr that the oscillating motions described by Ptolemy in the latitude theory could be accounted for by a combination of two circular motions. Once construed as such, the net effect of the motion of the Ṭūsī Couple could in addition account for the Ptolemaic statement regarding the inclined planes of the lower planets Mercury and Venus, which were supposed to seesaw in order to produce the latitudinal motion of these planets. The elegance and superiority of this solution of the oscillating motion, through a deployment of a Ṭūsī Couple, becomes very clear when we remember Ptolemy's alternative suggestion of having the tips of the diameter of the inclined plane be attached to two "small circles" so that he could achieve that seesawing motion — a motion that would, at the same time, destroy the longitudinal motion on account of the resulting wobbling necessitated by the "small circles." It was in that context that Ṭūsī felt that Ptolemy's speech was outside the craft of astronomy.

Instead Ṭūsī suggested that one could achieve a better seesawing effect, without having to accept the necessary result of wobbling. And in order to do that, Ṭūsī then produced a rudimentary construction of two small circles of his own, which were fitted in such a way that one of them rode on the circumference of the other, and had the tip of the diameter of the inclined plane attached to the circumference of the second circle as well. When the motions of the two circles were supposed to be in such a way that the one riding on the circumference moved at twice the speed as the other one and in the opposite direction, then the point at the very tip of the circumference of the riding circle, i.e. the tip of the diameter of the inclined plane, would end up oscillating along the joint diameter of the two circles as a result of their uniform circular motions. This produced at once an oscillating motion from two combined uniform circular motions, and allowed the tip of the circumference of the riding circle to oscillate along a straight line, thus keeping it from wobbling. The combined effect of Ṭūsī's two circles successfully produced a straight motion by combining two circular motions, a result that was to have tremendous effects on later astronomers.

About 13 years after he wrote the Taḥrīr (that is, around 1260 or 1261), Ṭūsī developed the idea further in his al-Tadhkira fī al-Hay'a (Memoir on Astronomy), and produced it in the form of a theorem, that is now called the Ṭūsī Couple (figure 4.7). He did reach the same conclusion a few years earlier when he included the same theorem in his Persian text Dhayl-i Mu'inīya, whose date of composition is still uncertain but has to be sometime between the publication of the Taḥrīr in 1247 and the Tadhkira in 1260/61, where the theorem is fully stated and proved.

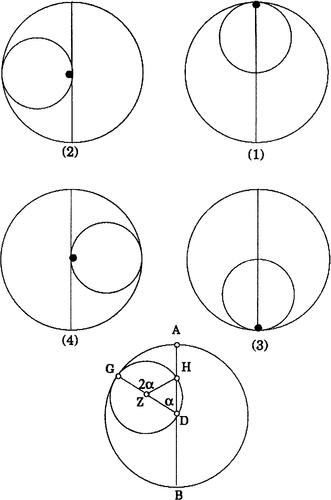
Figure 4.7

The Ṭūsī Couple. If two spheres such as AGB and GHD touched internally at point A, and if AGB's diameter was twice as large as that of GHD, and if the larger sphere moved uniformly in the direction indicated, and the smaller sphere moved in the opposite direction, at twice the speed, then point A would oscillate up and down the diameter of the larger sphere AB.

The theorem itself spoke of two spheres, instead of circles, one of them twice the size of the other, and placed in such a way that the smaller sphere was internally tangent to the bigger sphere as in figure 4.7 (1). Then Ṭūsī went on to prove that when the larger sphere moved uniformly at any speed, while the smaller one also moved uniformly, but in the opposite direction, at twice the speed, then the common point of tangency would end up oscillating along the diameter of the larger sphere.

Once he had generalized that theorem, he knew he had found a fecund theorem that could be used whenever one needed to produce linear motion as a result of combined circular motions. He then went on to produce, in the same Tadhkira, a formal proof for the theorem, as in figure 4.7, and later applied it to construct two of his alternative models: the lunar model and the model for the upper planets. In this fashion he then managed to solve the equant problem in the two respective Ptolemaic models for those planets.

The success of this theorem had widespread repercussions. It ended up being used by almost every serious astronomer that followed Ṭūsī, including the Renaissance astronomers such as Copernicus and his contemporaries, as we have already hinted and shall see again in more detail later on. In contrast to Copernicus however, the only place where Ṭūsī failed to apply his Couple, was in the case of the planet Mercury, whose behavior was quite challenging for Ṭūsī as we have already seen. When discussing that planet's motions in particular, Ṭūsī unambiguously declared that although he succeeded in solving the equant problem of the models of the moon and the upper planets, he was hoping to complete his task later on by solving the equant problem of Mercury, to which he had no new things to add at the time.

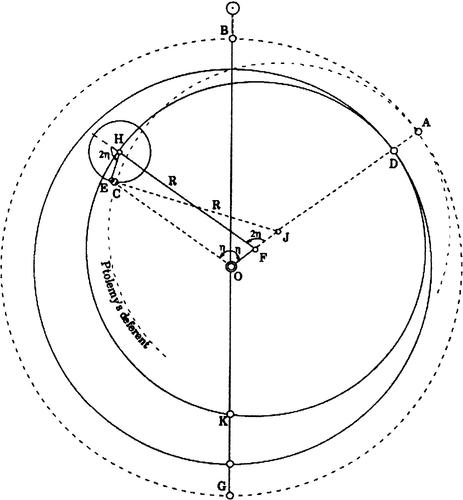
Figure 4.8

Shīrāzī's lunar model. By taking a new deferent whose center was halfway between the center of the world O and the center of the Ptolemaic deferent J, Shīrāzī compensated for that by introducing a new small epicycle, with center H, whose radius was equal to the same distance between the two centers of the respective deferents. By making the small epicycle move at the same speed as the new deferent, and in the same direction he managed to satisfy the conditions for 'Urḍī's Lemma, which could now be applied to lines HE and OF, thus making line EO always parallel to line HF, and making the epicycle center C appear to be moving around the center of the universe O.

Ṭūsī's student and colleague Quṭb al-Dīn al-Shīrāzī (d. 1311) made use of 'Urḍī's Lemma twice, once in developing his lunar model, and the other time when he adopted the same model for the upper planets as that of 'Urḍī In the lunar model (figure 4.8), he avoided the use of the Ptolemaic equant by bisecting the eccentricity of Ptolemy's deferent for the moon, and adjusting for it by positing a small circle at the circumference that satisfied the same conditions 'Urḍī's small circle satisfied in the case of the upper planets. That is, he allowed the small circle to move at the same speed as the deferent and in the same direction thus satisfying the condition of having two interior angles equal, and thus produced the parallel lines. The new arrangement, as suggested by Shīrāzī, made sure that the small circle, which moved uniformly around the center of its own deferent, also had the tip of its radius seem as if it was moving uniformly around the center of the world, which in turn was the observational condition Ptolemy's model satisfied. Thus by positing the Ptolemaic lunar epicycle at the tip of that radius the moon would then move around its own epicycle, but at the same time was seen to satisfy the same observational conditions it satisfied in Ptolemy's case.

In the case of the planet Mercury, Shīrāzī gave some nine models that could describe the motion of this planet. Those models are detailed in two of his most famous works, the Nihāyat al-idrāk fīdirāyat al-aflāk (the ultimate understanding regarding the comprehension of the spheres), and the Tuḥfa Shāhīya (the Royal Gift). In a still later work (Fa'altu fa-lā talum, meaning "I had to do it thus don't blame"), he signaled that seven of those models were faulty. Furthermore, one of the last two was also wrong. But the determination of which one was left unsaid so that Shīrāzī could test the intelligence of his readers, as he boldly claimed. The chosen model, which he finally claimed was the correct one, involved the use of two sets of the Ṭūsī Couple, arranged in such a way that it could successfully avoid the use of the Ptolemaic equant but preserved its effect and the conditions it entailed. That is, the final center of Mercury's epicycle seemed as if it moved around the point designated as the equant by Ptolemy, without ever having to have that motion come about as the product of uniform circular motions of any sphere around an axis that did not pass through its center.

Shīrāzī did not offer any new theorems, but obviously he benefited from his two contemporary astronomers, and deployed their theorems to the best of his abilities. One wonders why, for example, he opted to use 'Urḍī's model for the upper planets instead of the equally good model of Ṭūsī. But one has to also acknowledge that even if we cannot answer the question in the present circumstances, we can certainly affirm that Shīrāzī had at least two options and that he chose the one that deployed 'Urḍī's Lemma for his solution of the lunar model as well as the model of the upper planets, and reserved the double use of the Ṭūsī Couple for his Mercury model. One has to also acknowledge that Shīrāzī's double use of the Ṭūsī Couple for the planet Mercury, was in itself a significant step, not only because he succeeded where Ṭūsī had already declared failure, but because he seems to have put into wider circulation a novel idea, such as the double use of the Ṭūsī Couple, which was itself a remarkable departure from the accepted Ptolemaic astronomy when it used once. This remarkable achievement of Shīrāzī does not only put him at the forefront of the astronomers of his time, but allows us to see how novel ideas began to take hold in the scientific culture. They apparently succeeded when they began to be accepted and deployed by their contemporaries.

Shīrāzī insisted that one could begin to think of solving the observational behaviors of the planets by applying different mathematical techniques and producing more than one mathematical model. At the same time though, Shīrāzī was still under the impression that some mathematical solutions are more "true" than others. This attitude will become considerably important later on when we consider yet another conceptual shift as the one taken in the works of Shams al-Dīn al-Khafrī (d. 1550). Properly speaking, Shīrāzī's work lies at the beginning of a tradition that began to seek alternative mathematical solutions to the same physical problem. But at that early stage, the tradition still sought true mathematical solutions that could properly describe the motions of the planets. This tradition would not mature until the time of Khafrī. But just by seeking alternative mathematical solutions, and thus new ways of thinking about the problems, allows us to group Shīrāzī with 'Urḍī and Ṭūsī, who also created new shifts in the articulation of responses to Ptolemaic astronomy.

But because Shīrāzī also tried to group together a series of solutions which were offered by his predecessors, a series that he called uṣūl (principles/ hypotheses)[261], and which included such concepts as the eccentric versus the epicyclic models as two such principles, he can also be considered as the forerunner of the work of someone like Ibn al-Shāṭir (d. 1375), who came about half a century later, and who also used the solutions of his predecessors, and also globally called them uṣūl, as in his taṣḥīḥ al-uṣūl (correction of principles). In the case of Ibn al-Shāṭir, he too went beyond his predecessors and managed to succeed where they failed, again as in the case of the Mercury model that was correctly solved by Ibn al-Shāṭir when Ṭūsī had failed to accomplish that. But Ibn al-Shāṭir did more still, and deliberately carved new directions for his astronomy that also proved to be very productive for the Renaissance scientists.

There are several features that distinguish the works of this remarkable astronomer who apparently spent his professional life working as a timekeeper at the central Umayyad mosque of Damascus. Although we do not know much about the details of his job description as a muwaqqit (timekeeper), his works, both the extant as well as the non-extant, lead us to assume that in his "spare time" he indeed managed to develop one of the most successful attempts at overhauling Greek astronomy. Not only did Ibn al-Shāṭir profit from the astronomers who preceded him with their own attempts to reform Greek astronomy, but he managed to produce some remarkable conceptual shifts of his own in the way astronomy was to be perceived and practiced.

To start with, Ibn al-Shāṭir went back to the very foundations of astronomy and insisted on resolving the very first problem of Greek astronomy: a choice between an eccentric and an epicyclic model. To him that choice was definitely limited, for one could not in any way justify the use of eccentrics. To his mind eccentrics represented a clear violation of the Aristotelian principle of the centrality of the Earth, which up till his time at least made perfect sense within the overall universal cosmology of Aristotle. On that count he insisted that all of his models would adhere to these principles, and would be strictly geocentric. Furthermore, all of his models also shunned the eccentrics completely.

That left him with the problem of epicycles, which, as we have seen, had to be used in all the other planets except the sun. On that round he had some very original remarks to make, and as far as I can tell he was the first, and probably the only one, to insist on making them. First he made the general observation that the sizes of some of the fixed stars were in fact much larger than the sizes of the largest planetary epicycles. Second, when it came to the nature of the epicycles themselves, he tossed the ball back to Aristotle's court and to the court of his followers. Aristotle and his followers had insisted that the epicycles were not permissible because they would introduce a center of heaviness around which the sphere of the epicycle would move, and thus constitute an element of composition in the celestial domain, which was supposed by Aristotle to have been fully made of the simple element ether. Here Ibn al-Shāṭir wondered how could that be, when everyone knew that the stars which were carried by spheres that were made of the same element ether emitted a light we can all see, while no such light was emitted by the spheres themselves? How could one part of the sphere, where the star was located, emit all that light, while the other part remain dark, or transparent, and still be called a simple sphere? How could the sphere and the star that it carried be both made of the same simple element ether and have such divergent appearances? Once it was admitted that such phenomena existed, and there was no way to deny something that everyone could verify for himself, it became obvious to Ibn al-Shāṭir that at least the lower celestial spheres of Aristotle, by which the stars and the planets were supposed to be moved, had to admit some kind of composition. Only the uppermost sphere, the one beyond the eighth sphere that was only responsible for the daily motion of the whole, but carried no stars of its own, that sphere could remain as simple as Aristotle would want it to be.

That reasoning allowed him to conclude that the composition introduced by the planetary epicycles should at least be as acceptable as the composition, which was already implied by the existence of the fixed stars and planets that everyone could obviously see in the skies.

Having "solved" the problem of the epicycles in this fashion, he then went ahead and systematically banished all eccentric circles from his models. Instead he substituted epicycles for the eccentricities in each case, in a manner very similar to the application of the Apollonius theorem where the epicyclic model could easily replace the eccentric one. As a result, he then managed to produce a set of models that were all unified by their strict geocentrism. And in order to achieve that throughout he used a combination of two well-known principles that had already been used: the Apollonius equation and 'Urḍī's Lemma. The latter allowed him to adjust for the Ptolemaic equant by adding yet another epicycle, which was used by 'Urḍī in the model for the upper planets.

And because all his models were geocentric and used the same two "principles" to solve the equant problem, they also managed to expunge from Ptolemaic astronomy the variety of approaches that were adopted by Ptolemy in his quadripartite model structures—different models for the Sun, the Moon, the upper planets, and Mercury. With the exception of the Mercury model, all the other models of Ibn al-Shāṭir had identical constructions but whose representation of the planetary motions were simply manipulated by the sizes and speeds of the various epicycles he had to deploy. In the case of Mercury, he only introduced an additional use of the Ṭūsī Couple at the last step, but continued to use in it the two other principles just mentioned. This procedure was also followed some two centuries later by Copernicus, and for the same planet: Mercury.

One additional advantage resulted from this systematic use of geocentricity, which was to come in handy later on during the European Renaissance: the unification of all the Ptolemaic geocentric models under one structure that lent itself to the simple shift of the centrality of the universe from the Earth to the sun, thus producing heliocentrism, without having to make any changes in the rest of the models that accounted well for the Ptolemaic observations resulting from the equant. As we shall see later on, it may not have been entirely accidental that Copernicus ended up relying so heavily on the works of Ibn al-Shāṭir when he used, among other things, a lunar model that was identical to that of Ibn al-Shāṭir, and used the same Ṭūsī Couple, in the same fashion as was done by Ibn al-Shāṭir, in order to account for the motion of Mercury.

And despite common legends that claim that Copernicus was attempting to get rid of the equant,[262] by adopting Ibn al-Shāṭir's techniques, and just shifting the direction of the line that connected the sun to the Earth, he could in fact retain the observational value of the equant, without having to assume, as was done by Ptolemy, the existence of a sphere that could move uniformly around an axis that did not pass through its center.

In addition to the flexibility of Ibn al-Shāṭir's models, and his full control of the mathematics that allowed him to adjust his models so that they would fit the observations, Ibn al-Shāṭir also made another unprecedented step. He was the only one of the astronomers in the Islamic domain who seems to have devoted a whole book (Ta'līq al-arṣād, meaning Accounting for Observations) to this particular relationship between observations and the construction of predictive models that could satisfy those observations. The book seems to be unfortunately lost, and thus we may never know the extent of his theorizing in this regard. But it is extremely significant that he did undertake the writing of such a book.

And even if we have to assume that Ta'līq al-arṣād is lost to us, we still have some inkling about the methods and contents of that book from a few instances where such approaches have been followed in his surviving works, and in particular, in his Nihāyat al-sūl fī taṣḥīḥ al-uṣūl (The Final Quest Regarding the Rectification of [Astronomical] Principles). In this last book, we are explicitly told that Ibn al-Shāṭir had conducted his own observations in order to determine the apparent sizes of the two luminaries.[263] And we are also told that with those new results, which varied considerably from the values given by Ptolemy, he managed to construct a new model for the sun which was also at variance with the Ptolemaic model. In essence, this work demonstrates quite clearly Ibn al-Shāṭir's ability to construct theoretical models that were based on observational results, just as was done by Ptolemy, but without committing the inconsistencies of Ptolemy. It is in such instances that the centrality of Ibn al-Shāṭir's work can best be appreciated, and his relationship to Copernican astronomy can be better understood.

In all the previous Islamic responses to Greek astronomy one can detect a consistent tendency to solve the problems of that astronomy one problem at a time. From the prosneusis point, to the equant, and finally to the harmony between observations and predictive models, what the astronomers seemed to be doing was developing theorems and techniques that allowed them to reconstruct Ptolemaic astronomy along lines that would make that astronomy consistent with its own physical and cosmological presuppositions. What the predecessors of Khafrī seemed to be doing was trying to cleanse Ptolemy's astronomy from its faults, by using new mathematical techniques and theorems that were either unknown to Ptolemy or unnoticed by him. No one though seemed to think of the very act of mathematical theorizing itself and its relationship to the physical phenomena that were being described until Khafrī.

With Khafrī, Islamic astronomy moved to still newer territory. He was the first to begin thinking about the role of mathematical representation itself, the functionality of predictive models, and the relationship of all that to the actual physical phenomena. We had already noted the beginnings of this kind of thinking when we described Quṭb al-Dīn al-Shīrāzī's attempt at producing nine different models for the planet Mercury as a new step that marked the search for mathematical alternatives to the ones that were inherited from Ptolemy. But we also noted Shīrāzī's failure to pursue this line of thought when he seemed to have been still mired in the process of finding a unique solution, or say a unique representation, of the physical phenomenon that could be summed up in one true mathematical model. He spoke of such truths himself, for he was the one to alert his reader that of all the nine models he proposed for the planet Mercury in two of his books, seven were faulty by his own admission, while the eighth was left to the student to figure out its failings, and only the ninth was the true solution. So despite the fact that he was beginning to think that there were alternate mathematical techniques to describe the same physical phenomenon, he still thought that those techniques must climax in a unique solution that represented the truth of the matter.

With Qushjī (d. 1474) we can begin to see this trend pushed slightly forward. For although he must have known that Shīrāzī's model for Mercury solved the problems of the Ptolemaic model quite adequately, he still felt he could produce one more model that could do the same, which he did with his own new model. Was he thinking that his model, which relied entirely on 'Urḍī's Lemma to solve Mercury's equant problem, was only an alternative to that of Shīrāzī, which used only the Ṭūsī Couple, in the sense that it was a deployment of an alternative theorem to solve the same problem, or was he in fact thinking that the problem itself admitted multiple solutions? At this stage, we do not yet know. But from the fact that Qushjī's treatise on the Mercury model is a very short treatise devoted to this model only, one can presume that he was thinking of it as an alternative to Shīrāzī's and thus as just another way of thinking of mathematics.

With Khafrī the issue becomes very clear. In one full swoop he produced four different models for Mercury's motions, which he called wujūh (approaches), all of them accounting for the observations in exactly the same fashion, and none of them similar to the others in terms of its mathematical constructions. It is as if Khafrī had finally realized that there was a difference between two ways of thinking about Apollonius's theorem. On the one hand it could be thought of as representing two different cosmological solutions for the conflict between Aristotelian presuppositions and observations, and it can be thought of as two different mathematical ways of speaking about the variation of the solar speed with respect to an observer on Earth. It is the latter understanding that was finally realized by Khafrī's wujūh, for all his four models were mathematically equivalent in the same way the eccentric representation was mathematically equivalent to the epicyclic one. And although it is not stated in quite those terms, one could almost hear Khafrī say that the mathematical models he was devising were only different linguistic phrases used to describe the same phenomenon.

Seen as a tool, mathematics in the hands of Khafrī would become just another language of science, a tool to describe physical phenomena, and nowhere required to embody the truth or the correct representation, as was apparently thought by Shīrāzī before.[264] Mathematics became just as simple as describing a phenomenon with poetic language, with prose, or with mathematical figures, and as such the language itself can then be isolated from the phenomenon itself.

By focusing on the major shifts in astronomical thought that characterized the Islamic responses to Greek astronomy, it is now easy to see in hindsight the major features of these shifts. We can see how important it was to explore the full technical details of the most sophisticated Greek astronomical texts (the works of Ptolemy), not only to correct their mistakes, observational and otherwise, but also to investigate their presuppositions and the manner in which they related the observed phenomena to the methods of representation that allowed for the prediction of those phenomena. This close look at the foundations of those texts gave rise, as we have seen, to a series of Arabic texts written specifically for the purpose of critiquing the shortcomings of this imported Greek tradition. From istidrāk to shukūk, to straight forward rejection, all this full exposure left the Greek astronomical tradition in desperate need of reform.

The most important transformation that took place during this time was the shift from Ptolemy's instrumental approach to astronomy (which satisfied itself with the pragmatic success of the predictive features of the mathematical models) to a more theoretical approach which required that predictive results be consistent not only with the observations but also with the cosmological presuppositions of the observations themselves. In other words, in Islamic astronomy, it was no longer sufficient to say that a specific predictive mathematical model, such as that of Ptolemy, gave good results about the positions of the planets for a specific time. The new requirement was that the model itself should also be a consistent representation of the cosmological presuppositions of the universe, in addition to its accounting for the observations. If the universe was composed of combinations of spheres, and if those spheres were, by their very nature, supposed to move in uniform circular motions, then it was no longer acceptable to represent those spheres with mathematical models that deprived those spheres of their essential properties of sphericities and satisfy one's self by saying that they yielded good predictive results.

What seems to have happened during the confrontation between the receiving Islamic civilization and the imported Greek tradition, which we know was very closely watched by various sectors of the society, was to subject this incoming tradition to all sorts of exacting criteria before it was allowed to survive the cultural critique to which it was subjected. In that context, astronomy was no longer a discipline that only supplied good answers about the positions of the planets, or good enough answers for an astrologer to cast a horoscope. But with the Islamic religious aversion toward astrology itself and toward the craft in general, astronomy had to define itself as a discipline that went beyond that simple predictive feature and had to pose itself as raising questions of much greater relevance to a wider and larger world view that required exacting measures at every turn. The astronomer had to attend to all larger intellectual questions that had any bearing on his craft. In that concern the astronomer could no longer afford to seem as if he was satisfied with a confused picture of the universe, as long as he could achieve reliable results for astrological prognostications. Astronomy had to prove its usefulness to the new social and cultural environment within which it had to struggle. It could only do so by engaging the theoretical criticism of the very foundation of Greek astronomy.

In that context, one can then understand why no one could continue to tolerate two different visions of the nature of the universe that were in direct conflict with one another. One could not isolate the results presented in the Almagest, as mere computational and mathematical tools that could predict the positions of the planets at specific times, and say that they were irrelevant to the physical universe presented in the Planetary Hypotheses. To be fair, Ptolemy never really claimed that. On the contrary; throughout the Almagest he repeatedly hinted to the necessity of keeping the universe of the Planetary Hypotheses in mind. But at the same time he still went ahead and violated almost every feature of that universe by representing it with mathematical concepts that were totally divorced from their very mathematical properties. The example of the equant spoke directly to this point, where the spheres of the Planetary Hypotheses lost their very properties of spheres, if one were to represent them only in the manner in which they were represented in the Almagest.

With those fundamental oppositions, the job of the astronomer in the receiving Islamic culture became focused on those very issues of consistency between the vision of the Planetary Hypotheses and the representations of that vision in the Almagest. In the first phase of the response to the Greek astronomical tradition, the problem was perceived as a problem of sophisticating the techniques of representation, that is, the deployment of the same mathematics that was used by Ptolemy in order to reconfigure the representations so that they would be more faithful to the objects that they were representing. Someone like Abū 'Ubayd al-Jūzjānī (d. ca. 1070), the famous student of Avicenna (d. ca. 1037) did just that in his failed attempt to reform the representation of what later on became the equant problem. It took about two centuries to realize that Ptolemy's mathematics itself was inadequate, and that new mathematics had to be invented for the purpose.

The works of 'Urḍī and Ṭūsī ushered in the second, more important phase, when they spoke directly to that need of creating new mathematics. And each of them had a new theorem to add. Several astronomers, who used the newly enriched mathematics, and who also began to speculate about the various ways with which the physical phenomena could be mathematically represented, followed them. The nine attempts at representing the motions of the planet Mercury, which were devised by Ṭūsī's student and colleague Quṭb al-Dīn al-Shīrāzī, and the later attempt by 'Alā al-Dīn al-Qushjī to produce one more model for the motion of Mercury, fall in that category. This trend of re-defining mathematics as a language to describe the physical phenomena was to reach its climax with the works of Khafrī who finally gave concrete examples of four different mathematical models that described the motions of the planet Mercury, and yet were all exactly mathematically equivalent. In this fashion he could demonstrate, although never stated explicitly as such, that such physical phenomena did not yield unique mathematical solutions, but almost as many as the human imagination could conjure up, in exactly the same way a specific fact could be describe by an endless variations of the language.

With Ibn al-Shāṭir, the reorientation of astronomy took yet another turn, going back to the very cosmological foundations that were at the base of all phenomena as well as to the representations of those phenomena. Ibn al-Shāṭir ended up re-questioning the very use of the concept of eccentrics, and finding it cosmologically inconsistent with the cosmological foundations it was supposed to represent. When faced with the inevitable alternative of the epicycle, he insisted that such a tool be used despite the fact that the then current Aristotelian interpretation of his time thought of the epicycle as alien to the Aristotelian universe. Instead of giving up and pleading human imperfection, as was done by Ptolemy when the latter failed to find representations that were consistent with his own cosmological presuppositions, Ibn al-Shāṭir went back to the Aristotelian universe itself in order to criticize its inconsistencies and to point to the fact that such epicycles were, in a strict sense, consistent with an Aristotelian universe if the latter was properly understood.

Having banished all eccentrics in his representation of planetary motion, and having seen the essential similarities of all such motions in that they could all be represented by the same kind of model with a minor additional adjustment for the planet Mercury, Ibn al-Shāṭir went on to re-examine the relationship between the observed phenomenon and the mathematical models that were supposed to represent it. His readiness to adapt his mathematical models to match the observations speaks volumes about his priorities and about his ultimate re-definition of astronomy. To Ibn al-Shāṭir, astronomy was first and foremost a discipline that produced a systematic and accurate description of the behavior of the real universe around us. That description itself had to be a scientific mathematical representation that could only be a statement that described the reality of the observations.

Seeing these developments in Islamic astronomy in this fashion allows us to see how demanding the receiving culture was, and how its very demands required that its own scientific thought continued to be progressively defined and perfected according to the ever-changing criteria of precision and consistency that this culture imposed upon itself.